[A considerably expanded treatment of this material appears in Chapter 9 of the Energy and Human Ambitions on a Finite Planet (free) textbook.]
Today, we’re going to make the world less comfortable, in two easy steps that each of you can do at home. Step 1 shows how easy it is to account for the carbon dioxide excess in the atmosphere based on our cumulative use of fossil fuels. Step 2 bypasses intricacies of thermal radiation to put an approximate scale on the amount of heating we would expect the excess CO2 to produce. Serves 7 billion.
Climate Change in Context
I view climate change as a genuine challenge to the stability of our coexistence with the planet. But it is not my primary concern. A far more dangerous threat to the human endeavor is, in my mind, our reliance on finite resources and the difficulty our economic systems will have coping with a decline in the availability of cheap energy. That said, the issues are closely linked—through fossil fuels—and both benefit from a drive toward renewable resources.
Climate change is also important to the energy scene in that it provides a cautionary tale. Despite being a straightforward phenomenon (as this post will show), it has been incredibly easy for various interests to stir mud into the water and create substantial—and sometimes growing—doubt about the validity of climate change induced by human activity. Generally speaking, complex issues have enough loose threads that claws can easily grab hold and make a mess of things, distracting the public for decades about whether there is indeed a ball of yarn somewhere in the mess.
If we take decades to acknowledge en-masse that we have passed peak oil—whenever that happens (or happened)—and maybe really should make a long-term plan to mitigate the effects, we’re in trouble. Markets will exert powerful influences in the right direction, but perhaps not far enough in advance of a real problem to be effective, and the market “solutions” are almost certain to be expensive by today’s standards. In any case, the swirling controversy around climate change suggests that even if markets go into overdrive to address post-peak realities, there may not be much consensus as to why things are transpiring as they are. We are fond of narratives, and there will be an ample selection of compelling explanations from which to choose.
Inevitable Chemistry
Why do we loves our fossil fuels? It’s not for their visual aesthetics, their pleasant smell, or their enduring companionship. We only like them for their convenient, cheap energy. The energy is unleashed via combustion with oxygen. The chemical reactions for complete combustion of coal (represented here as straight-up carbon), natural gas (predominately methane), and gasoline (typified by octane) are:
C + O2 → CO2 + 7.9 kcal
1g 2.7g 3.7g
CH4 + 2O2 → 2H2O + CO2 + 13 kcal
1g 4g 2.25g 2.75g
C8H18 + 12.5O2 → 9H2O + 8CO2 + 11.5 kcal
1g 3.51g 1.42g 3.09g
Each reaction has been scaled for one gram of input fuel. Notice that all three produce about 3 g of CO2 for every gram of input fuel. This 3:1 ratio applies to any mass/weight measure you care to use, and is easy to remember. Example: fill a car’s gas tank with 30 kg (65 lb; 40 ℓ; 10 gal) of fuel, and out will pop about 90 kg of CO2. Could you lift that much?
Note that the 7.9 kcal derived from 1 g of carbon is a bit higher than for actual coal, which contains some volatiles and non-combustible junk. The real range is 3.6 kcal/g for lignite to 6.5 kcal/g for the ever-rarer anthracite. But for climate change, we’re only concerned with the amount of CO2 released per unit of energy derived. The mass of baggage in coal is irrelevant for our purposes (other than to bring the amount of CO2 released per gram of actual coal closer to the magic 3:1 ratio).
The CO2 intensity of each of these sources can be approximated by the amount of CO2 produced per kilocalorie of energy delivered. These work out to 0.47, 0.21, and 0.27 g/kcal for coal, gas, and oil, respectively, using the numbers above. I have seen this measure reported elsewhere as 0.39, 0.22, and 0.29 g/kcal—presumably accounting for the full suite of reactions in the real substance, rather than the principal ones considered here. Still, not too bad for a simple treatment (which is thematic of Do the Math posts).
Yearly Emissions
Fossil fuel energy accounts for roughly 85% of our 12 TW power production today, so let’s call it 10 TW from fossil fuels. This breaks up into approximately 3, 3, and 4 TW for coal, gas, and petroleum, respectively. This mixture results in a weighted carbon intensity of 0.31 g/kcal using our simple numbers, and 0.30 g/kcal using the alternate numbers. See—you worry too much: our simple numbers do just fine.
10 TW of fossil fuel power means 1013 J/s, or about 3×1020 Joules in a year (handy trick: one year is close to π×107 seconds), turning into 7.5×1016 kcal of fossil fuel energy used each year. Given our intensity, this results in the emission of 2.3×1013 kg of CO2 every year into our atmosphere. That’s 23 gigatons per year.
Big numbers can be hard to put in context. We have to compare to other relevant big numbers to make any sense of them. The atmosphere is vast and relevant, so we should compare to the total mass of the atmosphere.
Atmospheric Context
How massive is our atmosphere? The basic technique is simple: take the atmospheric surface pressure (measured in pounds per square inch, Pascals, whatever), and multiply by the surface area of the Earth to get the weight of the atmosphere. Atmospheric pressure lands near the convenient number of 100,000 Pascals (Newtons per square meter). Multiply by the surface area of Earth (4πR², where R = 6378 km, yielding 5×1014 m²) to get 5×1019 Newtons of weight. Weight is just mass times acceleration due to gravity, or 9.8 m/s², which for our purposes is as close to 10 m/s² as we might hope to get! So we end up with 5×1018 kg of atmosphere.
Adding an annual deposit of 23×1012 kg of CO2 to something that’s 5×1018 kg mean’s we’ll change the composition by 23/5 parts per million (ppm), or 4.6 ppm each year.
We have one more wrinkle to iron out before we compare our result to measurements of CO2, because the measurements are usually expressed as parts per million by volume and not by mass, as we have calculated. Roughly three quarters of the atmosphere is made of N2, at 28 g/mol, and one quarter O2, at 32 g/mol, for a weighted average around 29 g/mol. Meanwhile, CO2 weighs in at 44 g/mol. Gases come to equilibrium occupying a volume proportional to the number of molecules present (as counted in moles, for instance), so that the volume concentration of CO2 is less than the mass concentration by the ratio 29/44. This adjustment puts us at a yearly volume contribution of 3 ppm.
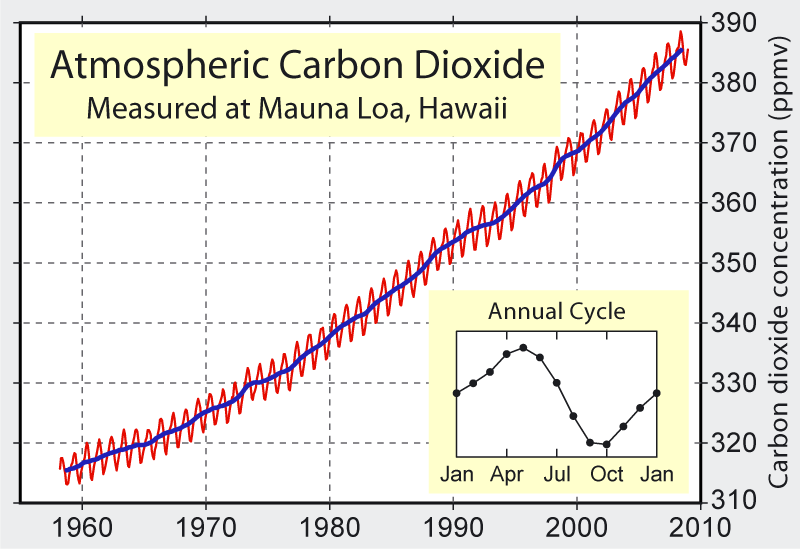
Atmospheric CO2 is rising around 1.9 ppm per year, up from a pre-industrial level of 280 ppm by volume. Source: WikiMedia Commons.
How does this compare to observation? The famous “Keeling curve”—representing measurements of atmospheric carbon dioxide begun in 1958 from Mauna Loa by professor Keeling of the Scripps Institution of Oceanography—lately shows an annual rise of about 1.9 ppm per year. I am told that about half the CO2 released into the atmosphere is promptly absorbed in the oceans (leading to acidification), so our numbers are entirely consistent.
In case the details of the calculation have clouded the simplicity of what we have done so far, here is a summary. Based on the principal chemical reactions that are non-negotiable if we want energy out of fossil fuels, we must get a predictable amount of CO2 out of the reaction. We know how much energy we get each year from fossil fuels, and the simple process of totaling up the resulting CO2 and relating this to the total atmosphere yielded an estimate right in line with observations. The origin of excess CO2 in our atmosphere is not mysterious.
Total Contribution to Date
It is also worth verifying that the total observed rise in atmospheric CO2 concentration is consistent with the total amount of fossil fuels we have burned to date. Pre-industrial CO2 measured about 280 ppm by volume, while today’s figure pushes 390 ppm. Is the excess 110 ppm reasonable?
To date, we have used about one trillion barrels of oil worldwide, or 140 gigatons (Gt). Meanwhile, scaling U.S. consumption suggests that the world has used about 200 Gt of coal, and 70 Gt of natural gas. The total is approximately 400 Gt of fossil fuels. Applying our handy 3:1 CO2 to fossil fuel input mass ratio, we expect about 1200 Gt of CO2 emission into the atmosphere, or about 600 Gt once half of it is slurped into the ocean. Recalling that the atmosphere is 5×1018 kg, or 5 million Gt, our 600 Gt of CO2 amounts to 120 ppm by mass, or 80 ppm by volume.
Does 80 ppm equal our target 110 ppm? You betcha! How can I violate mathematics this way (did I flunk it in school)? Because we have a variety of imprecise numbers feeding the problem: 50% absorption by the ocean, a round 400 Gt of fossil fuels to date, etc. Coming up bang on the target under such circumstances should arouse more suspicion than being a little off.
So the point is that both the yearly and total observed CO2 increases can be attributed to the amount of fossil fuels we have used historically. Turned the other way, we can produce a straightforward prediction of how much CO2 to expect in the atmosphere based on the simple chemistry of fossil fuel use—a prediction that is borne out by measurements.
A Blanket in the Atmosphere
Now part two of the recipe: how hot will the extra CO2 make us? Most physics students, once they learn about radiative heat transfer (affectionately called sigma-T-to-the-fourth), are tasked with calculating the Earth’s temperature in radiative equilibrium with the Sun. If done “correctly,” the answer is disappointingly cold because the greenhouse effect is not incorporated in the simple calculation.
The way it works is, the sun imbues a radiative flux of 1370 Watts per square meter at the position of the Earth. Given its radius of R = 6378 km, the Earth intercepts 1370 W/m² × πR² of the incident sunlight, since the Earth appears as a projected disk to the Sun. Most of this incident flux is absorbed in the oceans, land, atmosphere, and clouds, while the remainder is immediately reflected back to space so the aliens can see our planet. The absorbed part (70%) heats the earth surface environment and eventually is re-radiated to space as thermal infrared radiation, at wavelengths centered at about 10 microns—far beyond human vision (0.4 to 0.7 microns).
The law for thermal radiation is that a surface emits a total radiative power of A·σT4, where A is the surface area, σ=5.67×10−8 W/m²/K4 is the Stefan-Boltzmann constant, and T is the surface temperature in Kelvin. For instance, a patch of Earth at the average surface temperature of 288 K (15°C, or 59°F) emits 390 W/m² of infrared radiation. To figure out the temperature of the Earth, we demand that power in equals power out, and radiative transfer is the only game in town for getting heat on and off the Earth. If we did not have a balance between power in and power out, the Earth’s temperature would change until equilibrium was re-established. Hey—that’s what global warming is doing. But let’s not get ahead of ourselves…
While the Earth intercepts a column of light from the sun with area πR², the Earth has a surface area of 4πR² to radiate. Considering that 70% of the incoming sunlight is in play, we have an effective influx of 960 W/m² onto one quarter of the Earth’s surface area (why not half? much of the Sun-side of the Earth is tilted to the sun and does not receive direct, overhead sunlight). So the radiated part must work out to 240 W/m², which implies an effective temperature of 255 K, or a bone-chilling −18°C (about 0°F). Incidentally, if the Earth were black as coal, absorbing all incident solar radiation, the answer would have been a more satisfactory 279 K, or 6°C, but still colder than observed.
We know that 255 K is the wrong answer; off by 33°C. The discrepancy is the greenhouse effect, and to this we owe our comfort and our liquid oceans. The greenhouse gases absorb some of the outbound infrared radiation and re-radiate in all directions, sending some of the energy back toward Earth. Two-thirds of the effect (about 22°C) is from water vapor, about one-fifth (~7°C) is from carbon dioxide, and the remaining 15% is from a mix of other gases, including methane.
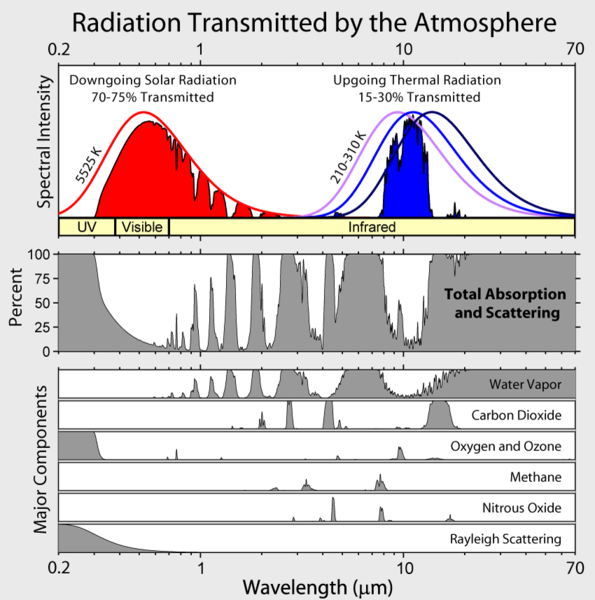
Incoming solar radiation (red) heats the Earth, which then radiates at thermal infrared wavelengths (blue). Most of the outgoing radiation is absorbed by water vapor, and some by CO2—especially at about 15 microns. Only the absorption features underneath the blue curve are relevant to the greenhouse phenomenon. Source: WikiMedia Commons.
One can see from the absorption figure that water vapor is responsible for the lion’s share of the infrared absorption at relevant wavelengths (under the blue curve), but that the CO2 absorption feature from 13–17 microns also eats some of the spectrum. A crude assessment tells me that the spectrally-weighted water absorption across the outgoing wavelength range is approximately three times as significant as the CO2 absorption feature, reassuringly in line with the 22:7 ratio.
Crudely speaking, if CO2 is responsible for 7 of the 33 degrees of the greenhouse effect, we can easily predict the equilibrium consequences of an increase in CO2. We have so far increased the concentration of CO2 from 280 ppm to 390 ppm, or about 40%. Since I have some ambiguity about whether the 7 K contribution to the surface temperature is based on the current CO2 concentration or the pre-industrial figure, we’ll look at it both ways and see it doesn’t matter much at this level of analysis. If CO2 increased the pre-industrial surface temperature by 7 K, then adding 40% more CO2 would increase the temperature by 7×0.4 = 2.8 K. If we instead say that 7 K is the current CO2 contribution, the associated increase is 7−7/1.4 = 2 K. Either way, the increase is in line with estimates of warming—though the system has a lag due to the heat capacity of oceans, slowing down the rate of temperature increase.
Keep in mind that these figures are based on today’s CO2 concentrations, not the impact of continuing to burn vast amounts of fossil fuels. We have spent about half our total conventional petroleum, and less than half of our total fossil fuel deposits. Thus the ultimate temperature climb could be well over 5 K (9°F) if we continue our practices unabated.
Using a linear relationship between CO2 and temperature change does not constitute a correct treatment, and would fail miserably for large adjustments to CO2 (like a factor of 2 or 3). But for the 40% change under consideration, it captures the direction and approximate magnitude of the effect reasonably well, which is the strength of the estimation approach: get the essential behavior without the burden of unnecessary complexity. A real treatment would acknowledge the saturated nature of the 15 micron absorption feature and use ΔT = C·ln(390/280), where ln() is the natural logarithm function, and C≈2.9–6.5 K according to the IPCC. This leads to an expected increase of 1–2 K at today’s excess concentration. But the point is already made without the fancy pants.
That Warm Fuzzy Feeling
This simple approach ignores a host of nonlinear feedbacks in the system that could either suppress or amplify the warming effect. But don’t let the wrinkles obscure the big picture. The chemistry and physics needed to understand that anthropogenic climate change is an expected phenomenon are not that hard. In fact, it would be difficult to make the case that burning fossil fuels on the scale that we have done should not increase CO2 concentrations by something on the order of 100 ppm total and recently 2 ppm/yr. Likewise, given the observation of increased CO2 levels in the atmosphere and our understanding of the role that CO2 plays in the thermal blanket, it would be hard to argue why the surface temperature should not rise by a few degrees Celsius.
But before anyone gets the impression that I claim this method offers irrefutable proof that climate change is real and expected, let me clarify that such a cursory analysis cannot constitute an airtight case. Leave that to the IPCC (which has, incidentally, concluded that anthropogenic global warming is an airtight case). But I find it personally persuasive, understanding the broad outline of the problem. Such simple analysis makes it easy to adopt a default position that climate change is real and expected. It would take a lot of convincing evidence of complex masking (conspiratorial?) mechanisms to destroy these pillars. Not impossible, but not easily done.
Now, did anyone save room for dessert?
Views: 3722
So the oceans (surface water?) consume half the CO2 we output, about 1.1 ppm/year of the total 3.0 ppm/year. That is more like a third, but close enough for physics.
Has anyone factored in the increase of CO2 generation by humans? The physical creature themselves. Population is like 7x larger now than it was 200 years ago. Also we need to factor in the other CO2 consumer, vegetation i.e. rain forests. Assuming the oceans have not changed volume in the last 10,000 years. We do have a lot more people and a lot less rain forests on this planet. Increase in production with decrease in consumption will create an excess. Either we plant more trees and stop deforestation (Hello Brazil) or we make reverse greenhouse machines that suck in the atmosphere and bind the CO2 to a liquid for storage. Somebody have a really big supply of NaOH??
It seems like there are two shortcomings here. First, because there is CO2 reabsorption and we aren’t making an estimate for how big that effect should be, basically any answer for human CO2 emissions will be treated as “verified” by this analysis. The observed CO2 increase is 50% as big as the human contribution to CO2? Then 50% must have been absorbed into the oceans. The observed CO2 increase is 5% as big as the human contribution to CO2? Then 95% must have been absorbed into the oceans. With feedback, the number can be bigger — the observed CO2 increase is 150% as big as the human contribution to CO2? Then there must be feedback mechanisms that amplify the warming effect. Therefore it seems like this analysis is unfalsifiable.
Second, one of the key numbers to determine the temperature effect of increased CO2 is just presented as fact with no background on how one might estimate its size one’s self. What method was used to determine that CO2’s effect is 7K of the 33K greenhouse effect?
Unfortunately, I find this article extremely unconvincing because it does not provide any calculations or estimations I can perform that would favor the greenhouse warming theory over other warming theories. Consider the possibility that warming is being induced by some external source (perhaps cosmic rays that affect Earth’s ability to radiate energy), which causes CO2 to be released in response — do any of these calculations do anything to cast doubt on that theory?
There will unfortunately be many who are unconvinced by ballpark analyses. But in case it helps:
A study by Sabine et al. (Science vol 305, p. 307, 2004) found that 48% of CO2 emissions are taken in by the ocean. I would feel rather unjustified in allowing large departures from this value.
As for where 7 K comes from, I did show where the 33 K total effect comes from. The diagram of atmospheric transmission gives an eyeball sense that water is dominant in the relevant mid-infrared band. CO2 is clearly the next in line. A crude integral tells me that the two have a roughly 3:1 absorptive influence on the thermal spectrum. I’m satisfied—nothing looks out of place here or requires a stretch.
You should also mention the fact that the amount of water vapour in the atmosphere is largely dependent on the surface temperature. If the temperature drops, water precipitates out of the air, cooling it further. If the temperature rises, water evaporates at a higher rate. So the CO2 humans put into the air raises the temperature a little bit. The increase in temperature raises the amount of water vapour in the atmosphere more, which increases the temperature further, etc. This is called climate forcing. It’s what allows a relatively small amount of CO2 to have a huge effect on the climate.
Right; my simple approach is feedback-free. Negative feedbacks would suppress the effect, while positive feedbacks would amplify them. The IPCC expects positive feedbacks to outweigh negative feedbacks, so my CO2-only warming would be a lower limit in this case. The water feedback you mention does happen, but another result is more clouds that reflect more light. I once did a simple calculation to indicate that clouds win (slightly) and keep the process from being a runaway. But at this level of complexity, simple calculations become less useful. Nature already shows us that the net effect is: more CO2 –> warmer. The post mainly shows why this should not be a surprising result.
Thanks for post (and the timeliness, considering rperry’s comments on the same day). Thinking about it in terms of “X energy burned equals Y CO2 released” makes it quite easy to understand what is going on.
I’m constantly amazed at the sheer lunacy of climate change deniers that can wholeheartedly disbelieve climate science, simply by saying “it can’t be true”. The effects of fossil fuels on the biosphere are indisputable; this analysis of CO2 in the atmosphere is a small part of the big picture.
It appears as if we are rushing headlong towards total annihilation of the Earth, and we’re all complicit in the dirty deed.
Hooray for people that educate others as to the inevitable results of our current living arrangement.
[unnecessary personal attack redacted by moderator…] I’m referring to the idea that little warming “annihilates” Earth. I have a sauna in my apartment and I can tell you with confidence that 3K does not annihilate anything.
—
To the original author: You write that “If CO2 increased the pre-industrial surface temperature by 7 K, then adding 40% more CO2 would increase the temperature by 7×0.4 = 2.8 K.”
I think you are mistaken in assuming linear dependency.
Your analogy to a sauna is not helpful in understanding how the world ecosystem will respond.
True that additional CO2 is not necessarily linear. But the direction is clear enough, and for the sake of estimation (sanity check) it’s not an inappropriate choice for a small (40%) increase. For example, quadratic would result in double the effect (7 K), while square root would result in 20% (1.3 K)—to give two examples of nonlinear effect. I’m not trying to dial in a robust prediction, but rather understand the general behavior. We understand the process of IR absorption by CO2 and a careful job fully accounts for the nonlinearities. This has not escaped attention of serious climate modelers. Rest assured that the basic story does not fall apart on replacing my linear stab with a proper radiative transfer treatment.
BTW, please read the discussion policy to understand the rules of the road.
Steve: All CO2 breathed out by humans was first absorbed from the atmosphere by plants. We’re carbon-neutral.
Ben: The amount of heat retained by CO2 can be tested in laboratory, and has been by many people, to the point where it’s simple accepted physics taught in schools. If you’re not convinced by other people’s claims you could do it yourself. That’s what’s great about science.
The amount absorbed by oceans is measurable by their increased acidity, and is consistent with the rest.
Your hypothesis seems to be that most human-emitted CO2 was absorbed by the oceans or other mechanisms, while cosmic rays or something unknown caused the planet to emit CO2 just over the past century or two in an amount that just happens to roughly match the amount emitted by humans, and for some reason that CO2 can’t be absorbed by the same mechanism that’s absorbing ours.
Forgive me if that seems less plausible than “the amount of carbon we’ve emitted pretty well matches the amount added to the air and oceans. QED.”
I guess it’s always possible to say that simple, obvious effects are not actually explained by their obvious causes, but rather by a multitude of things we know little or nothing about. But it’s seldom correct.
I think it’s great how a simple order of magnitude estimation is often more reassuring than complex (and at times convoluted) arguments and reports. This sort of simple sanity check is one of the most useful tools a lot of people don’t have, and it’s great that the reasoning is so well presented here. I’m glad you started this blog so that more people can learn how satisfying doing the math can be.
The only thing i wish you had done is calculate the increase in acidity of the ocean as well. Since you post is “climate change” it would of added to the overall post and not added that much work.
Cheers though i check this blog often as its a nice read.
Skeptical Science is running a series of posts which explain ocean acidification: http://www.skepticalscience.com/Mackie_OA_not_OK_post_0.html
Currently the series is up to part 16.
Ocean acidification can be calculated, but you need to know your chemistry in detail to attempt it. Perhaps this is one case where the back of the envelope is too small, even for a ballpark estimate. 🙂
I believe that the first order greenhouse effect is proportional to the logarithm of CO2, which is something you would need to take into account. In particular the forcing is something like 5 times the log (C/C0) for concentration C relative to reference concentration C0.
You should also read Ramanathan and Feng’s On avoiding dangerous anthropogenic interference with the climate system: Formidable challenges ahead on the cooling caused by aerosols (which will go away when the fossil fuels run out).
Tom,
Thanks for an excellent sketch.
Something you hint at but don’t expand upon: what sorts of temperature increases can we expect in the future? We know roughly how much fossil fuels are left, how much carbon they contain, and so on. We can project scenarios of how fast we’ll use them up.
Any chance you can…ah…do the math for us? What does your crystal ball say about how hot it’ll get?
Cheers,
b&
No crystal ball here, unfortunately. And my approach is only meant to capture the flavor of warming to assess its plausibility. But as other comments have noted, the linear approach I take to temperature—while capturing the direction and approximate magnitude of the effect—does not cut it for an accurate quantitative measure. Plus, I leave off the “toppings” of feedback effects. To get the best “predictions” we can make at present, see the range of possibilities reported in the 2007 IPCC Report. Their answer is 2–4°C (3.6–7.2°C) over 2000 levels by 2100 (0.6°C if we managed to cease CO2 emissions in the year 2000 or to quickly scrub out the atmosphere to this level and hold it there).
Predictions (extrapolations) are fraught with peril over such long terms. One scenario not addressed by IPCC is a societal crash brought on, for example, by energy resource limitations and resulting in drastically reduced use of fossil fuels. But this could be an even worse fate in many ways than unabated emissions and full-scale climate change. It’s also much further from the extrapolative path, so they are justified in playing it “safe” with their conservative scenarios—even if they prove to be wrong, ultimately.
[comment edited down by moderator]
There’s clearly a finite amount of hydrocarbons we can burn for energy production. Petroleum is roughly half gone, but there’s still lots of coal and natural gas. And there are resources like tar sands that would produce net energy, yes, but not a lot; personally, I don’t think it’ll ever be economically viable to go after them…but that’s a topic for another day.
Shouldn’t it be possible to estimate the total amount of CO2 that would be released were we to burn up all remaining reserves and use that as an upper bound?
Cheers,
b&
No point being precise about this estimate, but if we say we have used 25% of the hydrocarbons in the ground (some with very low EROEI, like oil shale), the current Co2 rise of 110 ppm becomes something like 440 ppm for a total of 720 ppm. According to the logarithmic prescription (now we’re talking large changes, so a linear treatment is bogus), we’d get anywhere from 2.9ln(720/280) = 2.7 K to 6.5ln(720/280) = 6.1 K. But at these levels, the feedback amendments are almost certainly not understood well enough to justify the decimal places, or even the most significant digit, for that matter.
Thanks!
So…in more familiar (to me) units, that’s a 5°F – 10°F rise if we suck the ground dry. That’s bad — really, really bad. But it’s an upper bound, and probably an unrealistic one, which helps. It’s not unprecedented in geologic history…but those changes are associated with mass extinctions.
It does give me reason to hope that we might not pollute ourselves to extinction, though clearly the only way to avoid that fate is to kick the hydrocarbon habit as fast as possible.
“If I were king,” I’d tax the hell out of petroleum and use the taxes to massively build out renewables. Renewables cost more than historical petroleum…which should tell us that we need to use some of that cheap petroleum now to bootstrap ourselves to pay for the renewables when the petroleum gets too expensive — otherwise, when petroleum really starts to run out, it’ll also be expensive, and we might not be able to afford either petroleum or renewables, leaving us paddle-less up the proverbial creek.
Cheers,
b&
Clearly the place to start for estimates of future warming is the IPCC’s AR4, but even just the WG1 report is an inch and a half thick. Another introduction could be James Hansen’s Target Atmospheric CO2: Where Should Humanity Aim? (pdf) which looks at things from the perspective of the paleontological record. CO2 probably reached 1500 ppmv about 50 Mya, and has decreased since, so nature has somewhat already run this experiment for us. What’s interesting is that Hansen et al find a climate sensitivity of about 6°C for a doubling of CO2, about twice the 2-4°C that the IPCC estimates. If you read AR4 carefully (e.g. Figure TS.5), or the paper On avoiding dangerous anthropogenic interference with the climate system by Ramanathan and Feng, you’ll see the reason for the discrepancy. The IPCC estimate is based upon air pollution (aerosols) continuing to provide a significant cooling effect. Aerosols exit the atmosphere in a year or two after entering the atmosphere whereas CO2 takes centuries to draw down. Thus if we cease burning fossil fuels, either because they run out or because we want to limit CO2 emissions, we will see a doubling of the temperature anomaly in a fairly short time frame. In a way, we are being lulled into a false sense of how bad things are by our pollution. This strengthens the rationale for doing something sooner rather than later.
It is also worth noting that Arrhenius looked at this problem over a century ago and got a similar order of magnitude…
Wow: he must be using the same physics I am using! 🙂