Since I was a teenager, I frequently heard stories that some guy had invented a car that could get 100 miles per gallon (MPG), but that powerful interests (often GM, Chevron, etc.) had bought rights to the idea and sat on it. We suckers were left to shell out major bucks for gasoline, when a solution was in hand and under wraps.
Leaving aside the notion that such a design would bring unbelievable prosperity to its holder (i.e., no real incentive to sit on it), let’s look at what physics says is possible.
We like cars because we can travel quickly from point A to point B. So let’s evaluate the energy requirements to make that journey at freeway speeds. We will use the somewhat awkward (although appropriate) speed of 67 m.p.h. because it conveniently maps to 30 meters per second. At these speeds, aerodynamic resistance is the dominant energy drain, so we will start by evaluating only this to get a lower bound on fuel efficiency, and find that we do a pretty good job!
What a Drag!
As long as your car is bigger than a dust grain, air resistance increases as the square of velocity. This is because in the car’s frame of reference, with an oncoming “wind,” the kinetic energy in the “wind” that the car disrupts depends on the square of the air velocity. If the car had no aerodynamic attributes (like a sheet of plywood face-on to the flow), it would basically rob the oncoming column of air of all its kinetic energy.
If the distance traveled is D, the car sweeps out a volume of air during its travel equal to the cross-sectional area of the car, A, times the travel distance, D. To make this more explicit, a car with a frontal area of 3 square meters (2 meters wide and 1.5 meters tall) traveling 25 miles (40 km) will impact a tube of air with base area 3 m2 and 40,000 meters long. The kinetic energy in this tube is ½mv², where m is the mass of the air involved. We already know that the volume of the air impacted is A·D, so its mass is just the volume times the density of air, which we will call ρ. At sea level, ρ=1.3 kg/m3 for air (for reference, water has a density of 1000 in these units).
Putting these together, a tragically un-aerodynamic car would see a drain of energy of Edrag=½(ρAD)v², where the term in parentheses is the mass of the air involved. A real car has better aerodynamic performance than a piece of plywood, so we include a term called the drag coefficient, cD, and the energy expended on fighting air for the journey becomes Edrag=½cDρADv². The drag coefficient for cars ranges from 0.25 for a Prius to numbers like 0.5–0.6 for SUVs and pickup trucks. Loads of sedans come in around cD=0.3, so we’ll use that number for the present analysis.
Heat Engines and Gasoline
We, of course, get the energy to fight air resistance by burning gasoline in our engines. Automobile engines constitute heat engines, whose thermodynamic efficiency is bounded by limitations on entropy to be no more than 100×(Th − Tc)/Th percent, where Th and Tc are the hot and “cold” temperatures (in Kelvin) that the engine operates between. This, plus practical limitations, puts most automobile engines in the range of 15–25%. If we could achieve an ideal heat engine operating between the volume-averaged explosion temperature of around 1200°K (my guess) and the ambient 300°K, we’d have an efficiency of 75%. But the exhaust manifold—acting as the “cold” temperature for the cylinder—is much warmer than ambient temperature, and the cylinder walls moderate the effective hot temperature to reduce the theoretically achievable efficiency. A turbo-pump can use the hot exhaust to recover some of the lost energy flow and achieve better performance.
Gasoline delivers 36.6 kWh (132 MJ) per gallon. Used at 20% efficiency, this translates to Edeliv=26 MJ (megajoules) of energy actually delivered to the drive train per gallon burned.
Our Calculated Mileage
We are now in a position to calculate how many miles we can expect to travel per gallon of gasoline. Setting the energy delivered by a gallon of gas equal to the energy required to overcome air resistance: Edeliv=½cDρADv² and solving for D using the values sprinkled above, we find that D=50,000 m, or 50 km, or 31 miles. So our hypothetical air-resistance-only car gets 31 MPG at freeway speeds. Seems completely reasonable. We’ll adjust this and all other air-resistance-only calculations later for rolling resistance.
Pushing Limits
What could we do to improve mileage? The simplest option is to slow down. The quadratic dependence on velocity is striking, and this is where the biggest, easiest gains may be had. But we like to engineer our way to a solution, not change behaviors. So the knobs are: engine efficiency; coefficient of drag; and frontal area. The best, weird-looking concept cars achieve drag coefficients around 0.15. If we were willing to live with trout-shaped cars, we might get cD down to a bit less than 0.1. Diesel engines operate at a higher temperature and get better thermodynamic efficiency. The best of the best (in train locomotives and large ships) get 50%. For a gasoline engine made from steel, 30% would be a stretch. Decreasing frontal area is not usually compatible with our need to transport multiple people. If we were willing to sit single-file, we could do the trout shape with low frontal area. We might even stop hearing “stop touching me!” from the back seat. I will point out that trout cars would be a real pain to parallel park, necessarily being pretty long—with much of the length in a tail section that is too narrow to be of much use.
 As a fantastical example, using A=1.5 m², cD=0.1, and engine efficiency at 50%, we get the astounding fuel economy number of 466 MPG. But you’re not going to get a locomotive-performance engine in a 1.5 square meter trout-car. A more practical set of limits given our behavioral and aesthetic preferences might be A=2.5 m², cD=0.2, and 30% engine efficiency. This puts us at 84 MPG. Not a bad place to be, but shy of the magic 100 MPG. And even this is not a snap: note that we are nowhere close to this mark at present.
As a fantastical example, using A=1.5 m², cD=0.1, and engine efficiency at 50%, we get the astounding fuel economy number of 466 MPG. But you’re not going to get a locomotive-performance engine in a 1.5 square meter trout-car. A more practical set of limits given our behavioral and aesthetic preferences might be A=2.5 m², cD=0.2, and 30% engine efficiency. This puts us at 84 MPG. Not a bad place to be, but shy of the magic 100 MPG. And even this is not a snap: note that we are nowhere close to this mark at present.
How does the Prius today get a fuel economy in the low 50’s? The drag coefficient is on the low side, at 0.25. The area is small-ish—I estimate 2.5 m², and the big trick is that the engine can be optimized for freeway speeds since the battery can assist acceleration at lower speeds. Traditional cars sacrifice freeway efficiency for the get-up-and-go performance that is so important in test drives. If I use 25% engine efficiency with the aforementioned values, I get 56 MPG.
What About Rolling Resistance?
We have so far neglected rolling resistance (mainly from tires) in this analysis, primarily to keep things simple while capturing the dominant contributor to fuel economy at freeway speeds. At a rolling friction coefficient of 0.01, a 1 ton car (1000 kg; 10,000 Newtons) requires 100 Newtons of force to push along—independent of velocity. This effect alone (e.g., driving in a vacuum) would result in a limit of 160 MPG at a 20% engine efficiency. At 30 m/s (67 m.p.h.) in air, factoring in rolling resistance: our 31 MPG sedan becomes 26 MPG; our 56 MPG Prius becomes 45 MPG; our absurd locomotive trout car at 466 MPG becomes 220 MPG; and our “realistic” 84 MPG car now gets 63 MPG.
But keep in mind that these numbers are not to be taken too literally. We made plenty of round-number estimates for frontal area, engine efficiency, etc. The numbers are reasonable and give us a framework for understanding approximate limits. So I don’t want to hear anyone speaking of 63 MPG as a meaningful hard limit. It’s ballpark. It’s useful. Lots of details could be added to the analysis, but we’ve already captured the essence of the problem.
The Point of it All
The purpose of this post is to illustrate that fuel efficiency on the freeway is not mysterious. It’s the air, stupid. We have few knobs to turn, and are limited in how much more we can turn them. The biggest disappointment, perhaps, is the typical 20% performance of our engines. Naïvely, this suggests a factor of five potential gain. But as long as we’re making fireballs in our cylinders, we’re limited by harsh thermodynamic realities. A future post will deal with the potential of electric cars. We’ll have to abandon gallons as a measure (and when we do, we should also flip the measure to be energy per distance, opposite our familiar MPG).
Views: 11563
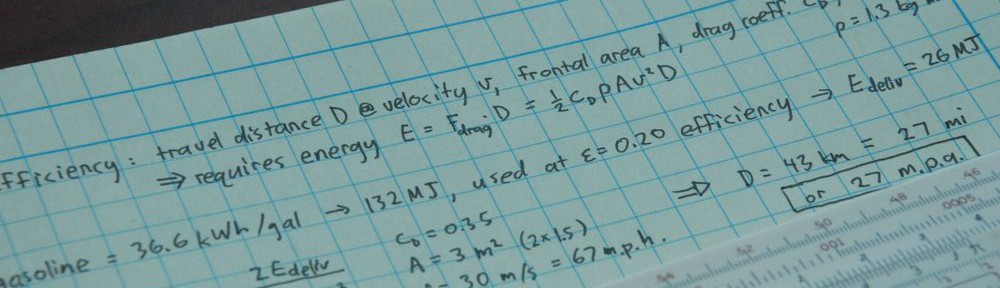
Great blog!!! Please, continue.
Thanks for the great article. Looking forward to the next one.
Thanks for the encouragement (and you too, Marcel). I came out of the gate fast and hard, but since I am coordinating with the Energy Bulletin for a weekly series, I may hold off a bit and let them catch up. But fear not: I have loads of post ideas I am raring to publish, so definitely stay tuned!
Look forward your thoughts and calculations on electric cars as many people seem to believe that we should not worry about gas prices by simply going electric (made in China, of course). It seems to me that energy density and power, in addition to air drag, of course, puts some fundamental limitations to battery capacity and recharge rate.
In case you’re seeking suggestions/ideas, it would be great to see someone with your level of mathematical rigor discuss the energy requirements of a (permanent, sustainable) human colonization of space. By that I mean, the energy bill for launching, constructing, and maneuvering the people and infrastructure necessary. My thought is if we’re entering a paradigm of an energy plateau or decline, the real-world cost of such a venture is only increasing from here on out, making it become less feasible in the future, not more feasible.
You forgot about rolling resistance, i.e. tire friction.
You’re right: rolling resistance is a contribution, although at freeway speeds, it is sub-dominant compared to air resistance. A quick estimate by two methods indicates a rolling frictional force between 50 and 100 Newtons, compared to about 500 Newtons from the air. So a 10 to 20% effect, by the quick calcs. So yes, it is an important piece. And it reinforces the conclusion that 100 MPG on gasoline is a goal we are unlikely to see achieved. I didn’t want to bog down the blog with a “equal time” treatment of a small effect, but perhaps I could revise with a paragraph to address the magnitude of the effect…
great website and great blog but . . .
I agree that the magic 100 mpg figure is energetically a long ways away from 80 mpg.
However, you make it sound too hard to get partway. Many manufacturers in europe offer normal cars (Ford, VW, Audi etc) with offical mpg readings of 70-75mpg. These are not hybrids or premium cars, and sell at normal prices.
Given the hideous mpg figures of most US cars, this would be almost as big an advance as getting 100mpg. And its not any more complicated to achieve than loading up a ship full of normal cars and taking them to the US.
Excellent point: thanks for contributing this. And in the more sensible European metric of liters/100-km, the difference between 70 and 100 mpg is not as large as it seems. And I certainly would not want this post to discourage all-out efforts to improve fuel efficiency. Hopefully it has some bit of opposite effect in letting Americans know that we can still do much better (though cannot improve without limit). Or maybe it’s another strike against gasoline, given the crummy heat engine performance.
“trout cars would be a real pain to parallel park, necessarily being pretty long—with much of the length in a tail section that is too narrow to be of much use.”
If the uselessly-narrow tail section were to comprise no more than half the total length, perhaps it could hinge upward (or sideways) so as to be stowed parallel to the rest of the vehicle instead of in-line. Alternatively, perhaps it could be made of telescoping sections; it might not even need to be deployed at in-town speeds.
What about weight? Weight is by far the most important consideration when it comes to MPG. Drag is important sure, but as long as your goal is to move obese amerikans in 6000pd vehicles short distances at 75mph or more, drag is not really going to play much of a roll. 100MPG is perfectly doable, but the resulting vehicle would not be to the likeing of what North Americans have been programmed by decades of relentless oil-auto cartel advertising to accept as ‘normal’.
And keeping with the theme of your posts on economic and energy growth, even if every single N.A. had a 100MPG vehicle today, how many years would that shift the Global peak? If at all. No matter how great the MPG of a gas-burner. at some point, the idea that everyone in society would and should have a gas-burner in there single family homes garage would have to be abandoned. This is why I think amerikans delude themselves when they annouce that 60mpg or somesuch will have anything more than a cosmetic effect on Peaking oil production or even pollution. The damage has allready been done.
http://www.go60mpg.org/
Weight is certainly an important factor in accelerating the car. So in stop-and-go traffic it can indeed dominate. Once you’re up to speed on a freeway for a long trip, it’s a story of drag, and the weight has little impact. The weight does impact the rolling resistance (proportional to gross weight), but this is a sub-dominant term at freeway speeds. I should perhaps follow up at some point with an analysis of the slow, high-traffic case…
Now you’re getting somewhere! I think DC is correct about 100 mpg being possible, theoretically, and you’re both correct that it would be a vehicle that no narcissistic developed-, or developing-, country’s citizens would “want.” (Of course, most Americans probably would not be able to get in one, either!) Furthermore, as you both allude, that type of mileage would ONLY apply on an “open” and relatively “level” highway (or downhill). Regardless, no matter how much “better” fuel consumption becomes, Jevon’s Paradox will remain a fly in the ointment and no “meaningful” result will be achieved.
My vehicle, like many others, has an on-board computer that will display my “average” fuel consumption, an approximate “range” based on fuel in the tank AND will report my instantaneous mileage. In that latter mode several “unknown” or “misunderstood” aspects of “mileage” become apparent. First, every “complete stop” one makes has an enormous impact on average mileage, much like getting just one “F” impacts an otherwise stellar GPA. Overcoming the inertia of the car at rest (remember Newton?) is 1) dependent on vehicle weight, 2) inclination of the road and 3) the “weight” of the driver’s foot (though to a lesser degree… if you pay attention). Moreover, the astute observer will notice that traveling at a slower cruise speed makes little if any difference on mileage. I see the same instantaneous mileage weather I’m cruising at 35 mph or 60 mph. (Yes, “drag” will become a more significant factor at speeds in excess of most “legal limits.”) Therefore, anyone who commutes more than a few miles and/or “has to” make more than few stops during that commute will NEVER see even the lowest “certified” EPA mileage for their vehicle. Please, don’t believe me! Set the appropriate mode on your vehicle’s computer, get a “copilot” with stopwatch to monitor and record those “instant” mileage numbers and their duration and gain some awareness. Sapere aude!
Beyond that, so far I’ll say “Nicely done, Dr. Murphy.” I do look forward to reading more. (I’ve read all you have here so far.) However, just 2 more things. I wonder who you perceive or hope(?) to have as an audience? Your “back-of-the-envelope” calculations are great for “lay people” but I doubt many of “them” will understand your logarithmic graphs and confuse your meaning. (Hell, most won’t grasp exponential curves, either, so it probably doesn’t matter.) Lastly, I find your “optimism” about the future admirable but unfounded. If you’d like to have your rose-tinted glasses “cracked” (if not shattered), please send me note. I’d also be delighted to hear/discuss more about your testing of relativity. That could be a “hoot!” 😀
Good comments. Having instantaneous and average mileage in a car is a huge boon, and you can definitely learn a lot. The few times I’ve had occasion to experiment (on a flat, straight road in NM), I have found an optimum of about 45 mph. Engine efficiency as a function of RPM (therefore speed/gearing) keeps it from being a straight-up drag plus rolling resistance problem.
As for intended audience, I want to share the power of simple estimation with whoever is interested and can take it in. For those lacking the ability to follow every nit, at least I hope they can skim to the point where I arrive at a conclusion, and they can at least appreciate the flavor of the method used to get there.
Regarding optimism, this is a tricky subject. I see lots of failure modes and a few successful trajectories. I am committed to doing all I can to aim for one of the smooth rides to the future, even if unlikely, on balance. If I had no shred of optimism, I would not have deemed it worth the effort to start a blog that points out the challenges.
On instantaneous and average mileage displays in cars: Does anybody know how they measure it?
I thought flow sensors would be (too) expensive and are tricky because common pump design pumps most of volume in circles.
Just measuring torque and calculate the mileage based on some calibrated table and interpolation is probably the cheapest (and every dollar counts in the car industry) but it would be questionable what one can learn from this.
I always look at this display with skepticism.
Daniel, (above or below this point?)
When I was taking classes in “Fluid Power” (included both hydraulics and pneumatics) ~25 yrs ago I recall using several flow-rate sensors of varying size that were relatively easy to incorporate in any experiment. I don’t “know” what they cost but judging from their design and construction I can’t imagine they were too expensive, even then. After all, the fuel-line (or whatever conduit for whatever “fluid”) is a known and unchanging diameter so all that is really required is a measurement of the fluid velocity through it and a calculation that wouldn’t even be noticed by an 8080 processor. Another simple calculation using that result and the velocity at a given “instant” and voila, “instantaneous mpg.” I wouldn’t make any bets regarding any value that was “too precise” but those indicators probably give a fairly reasonable approximation. Getting the “average economy” over a period of days/weeks/months is actually even easier.
Oops! My fingers forgot to type a word. Third from last sentence should read… Another simple calculation using that result and the VEHICLE’S velocity at a given “instant” and voila, “instantaneous mpg.”
Regarding the calculation of MPG by your car…
Fuel injectors are rated in CC’s for a given fuel pressure (typically 43psi), the instantaneous and running MPG numbers spouted off by your cars computer are based on the calibration of the fuel injectors. Every time an injector fires it is fired for a specific “pulse width”, the injector size divided by the width of the pulse gives you the amount of fuel injected by that injector for that particular revolution of the engine. Add the many thousands of these per minute up and do a bit more math and you have your various MPG figures.
A “flow sensor” would be useless for this task in most automotive fuel systems, as most of those systems move far far more fuel than is needed through the system at any given time and return unneeded fuel to the tank in a cycle.
Readers might find this paper interesting:
Locomotion: Dealing with friction, 1998 (Radhakrishnan V, (PNAS) Proc Natl Acad Sci 95:5448-5455)
http://www.pnas.org/content/95/10/5448.full.pdf+html
I’ve achieved 80mpg in two different standard Skoda Octavia diesels – for your American readers, that’s a VW Jetta / Bora equivalent. One was an old pdi engined saloon, on a solo 10 mile run along country lanes at 40mph. The other was an estate / station wagon / combi, doing 57mph on a freeway, cruising at a sensible stopping distance behind a truck. Both occasions were in high ambient temperatures.
Roll on the gently driven PHEV hybrid diesel. That’ll do 100 mpg for sure… But the most important device of all is the fuel efficiency readout on the dashboard. That, and the $10 gallon we Brits pay, are what change the behaviour…
Great info—thanks! Yes, there are some good tricks. Slowing down offers a big win, as does drafting behind a truck (actually improves truck’s economy too!). You mention the heat, which is important because air density scales like pressure divided by temperature (in Kelvin), so warm is less dense and lower drag. Also high altitude helps because pressure is lower. The best mileage I have gotten in my vehicle has been on the high plains of Colorado and Wyoming. In such places, air density can be lower than the sea level value (used in my analysis) by about 15%.
Can you compare British MPG and US MPG? They are different volumes – Is this Beagle2 happening here?
http://www.wolframalpha.com/input/?i=compare+uk+gallon+vs+american+gallon
An imperial gallon is 20% larger than a U.S. gallon, so for instance 93 MPG imperial (reported by Ralph w) turns into 78 MPG U.S. Note that this feat was accomplished with a small car at 55 m.p.h. drafting behind a truck.
And I’ve done 93mpg in my Skoda Fabia – a smaller car – under similar driving conditions. Of course those are UK mpg.
In the hands of an expert the same car has returned upto 126mpg (UK) over a round trip of 2000km. That is a genuine 100mpg (US) car, on sale today, in Europe. Seats 5 and reasonable luggage space. Just learn to drive slowly and very smoothly…
I had wondered about the heat, thought it was to do with the air entering the engine… Similar jorney in the dark dead of winter with pax and three Irish Setters on board yielded a mere 60mpg.
Hi Ralph… Fancy bumping into you here… 93mpg… I’m Not Worthy! But mine’s not the Greenline, more greased weasel, nearly vRS, and I also carry the optional spare tyre!
The instantaneous and average mileage are calculated from the fuel injector opening times. The car’s ECU fires the fuel injectors for a certain amount of ms and knows how much fuel flows through during that time.
Any car with electronic fuel injection (pretty much every car made in the last 20-25 years and many before that) has all the information to show the mileage.
Powerful analysis. Thank you!
Here’s my own take…
http://www.deathbycar.info/2011/08/mpg-trick/
Nice article. Can you do the energy cost limits on a plane journey some time? I keep hearing how air travel is going to be fine because the efficiency will double or triple, and I’m thinking surely planes are over 50% efficient already.
What I find most interesting is that the super-low-drag car you describe really does describe a fish. Given that the lower the drag a fish experiences the less energy it has to expend to move the shape makes sense.
In the early 1970s, in Kansas, a device was marketed that touted mileage increases in the 40mpg to 50mpg range with a standard carburetor engine (4x to 5x the common mileage of the landsharks of the day). It supposedly used exhaust heat to gasify the liquid fuel and with some carb tweaks allowed the phenomenal mileage.
News of this hit the papers, sales started to spiral up, then the next thing we heard the Attorney General was investigating this device and its inventor. Within a month it was declared unsafe and pulled from the market.
Sadly my sporadic online research has never yielded results on the device.
My questions:
Would something like this actually work, or was it voodoo BS?
Would the device at this point (assuming it did work) actually offer any advantages over a modern fuel injected engine?
Thanks for what looks to be an *extremely* interesting blog and for reading in.
ML
Was most likely a scam, you can find all sorts of these wonder devices on the web today. The thing that stands out about them is they almost to a one, do not have an actual product you can buy and install in your gas-burning trash-bin, but they usually offer ‘memberships’ or some nebulous plan to extract money from suckers while not acually delivering anything of even remote value.
Boosting mpg is not rocket science. Weight\mass is what its all about. Extermely light, low center of gravity, low speed! is what will get you there. Current cars will never be ‘efficent’ since they are expressly designed *not* to be. Heavy, overbuilt, filled with complex crap that is neither helpful nor necessary ie GPS, mp3 players voice-activated crappola, power, well..everything, camera systems, you name it. Sinply pouring in some wonder additive or adding more devices to the allready overbuilt ICE engines of today does not increase mileage, it would only decrease it. More weight, more mass, more complexity added to a system in every case will reduce efficency, not increase it. You may see some improvement in some other metric, but at the end of the day, the only way to make a gas-burner more ‘efficent’ is to make it lighter and slower and less complex.
Aptera.com