This is a quick update regarding the first plot shown in the galactic scale energy post. A reader, Chris, called attention to the obvious departure from exponential growth in recent decades. The post required turning a blind eye to many practical issues (like population saturation) in order to entertain indefinite growth, serving to highlight the absurdity of the notion. But Chris goaded me into paying more attention to the departure from the exponential track in the actual data, and here are the results of a logistic approach.
A logistic function looks like an exponential in its early stages, but goes through a linear inflection and ultimately levels out to a constant value. Sometimes called sigmoids (for their S-shapes), or population curves, logistic curves show up frequently in scenarios where initially exponential growth is capped by limited resources.
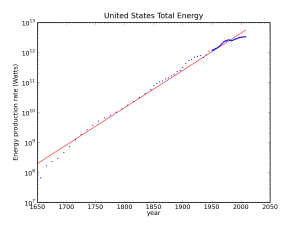
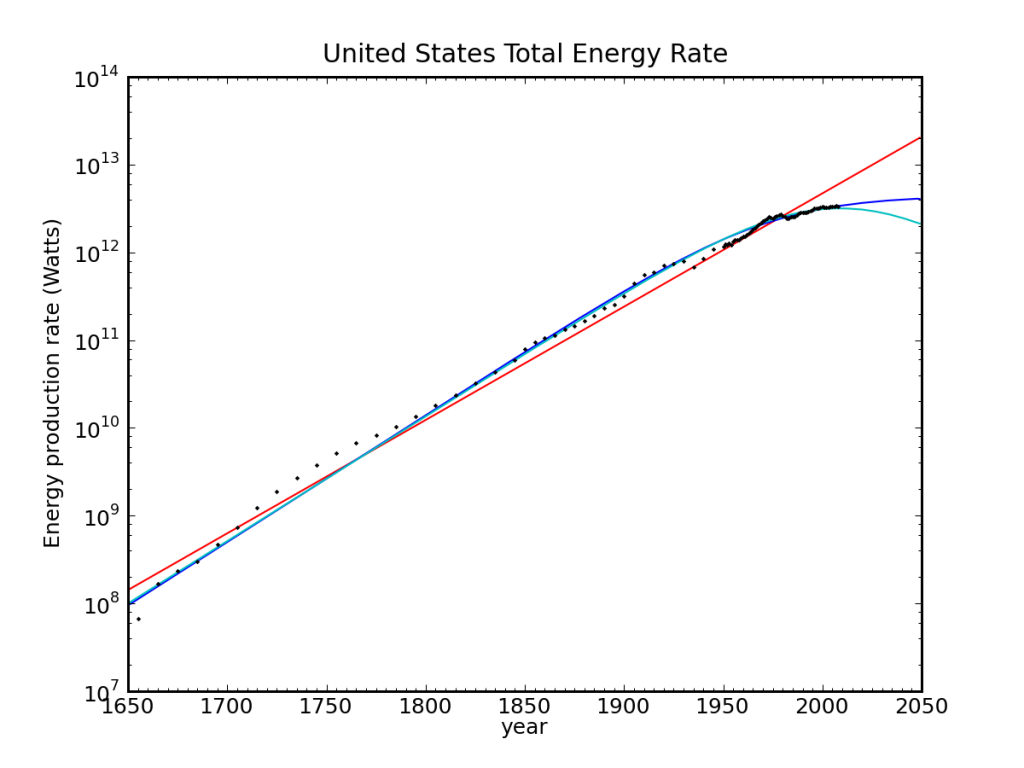
The red exponential function in the original plot (exponentials are straight lines in plots where the vertical axis is logarithmic) fits the U.S. energy growth curve relatively well for centuries, before peeling off starting around 1980. Let’s apply a logistic curve and see what happens.
But first, there are two ways to apply a logistic curve in this scenario. We will call the exponential trajectory the “infinite growth” scenario. A logistic curve that ultimately flattens out to mean that we’re producing a constant energy output each year might be called the “sustainable” scenario, because we would have to use renewable inputs (transitioning away from finite resources) to carry this out. On the other hand, to the extent that our meteoric energy trajectory is a mere reflection of a finite resource like fossil fuels, then the logistic function would apply to the total energy extracted, and it is the cumulative energy used that flattens out. In this case, the slope of the logistic curve is the rate at which we produce energy each year. If we did not adapt to renewable inputs, our trajectory would roll over and decline to (essentially) zero in the future. We can call this the “finite resource” scenario.
In this semi-logarithmic plot, the black points are the U.S. data, as posted previously (with references). The red curve is the exponential fit, corresponding to 3% growth per year. The blue curve is the “sustainable” trajectory, and the cyan curve is the “finite resources” fit. These are not fit by eye, but by an objective least-squares parameter estimation. The “sustainable” curve levels out at 4.4 TW (we are at 3 TW today), and has an inflection (halfway point) in 1973. The “finite resource” fit peaks in 2009 just above 3.1 TW. Both logistic curves do a far better job fitting the data in recent times than does the exponential. But isn’t it remarkable that we cannot readily distinguish the sustainable from the power-down scenario based on the current data? This is a severe warning for those who wish to extrapolate the past into the future. The math is happy with either, at this point.
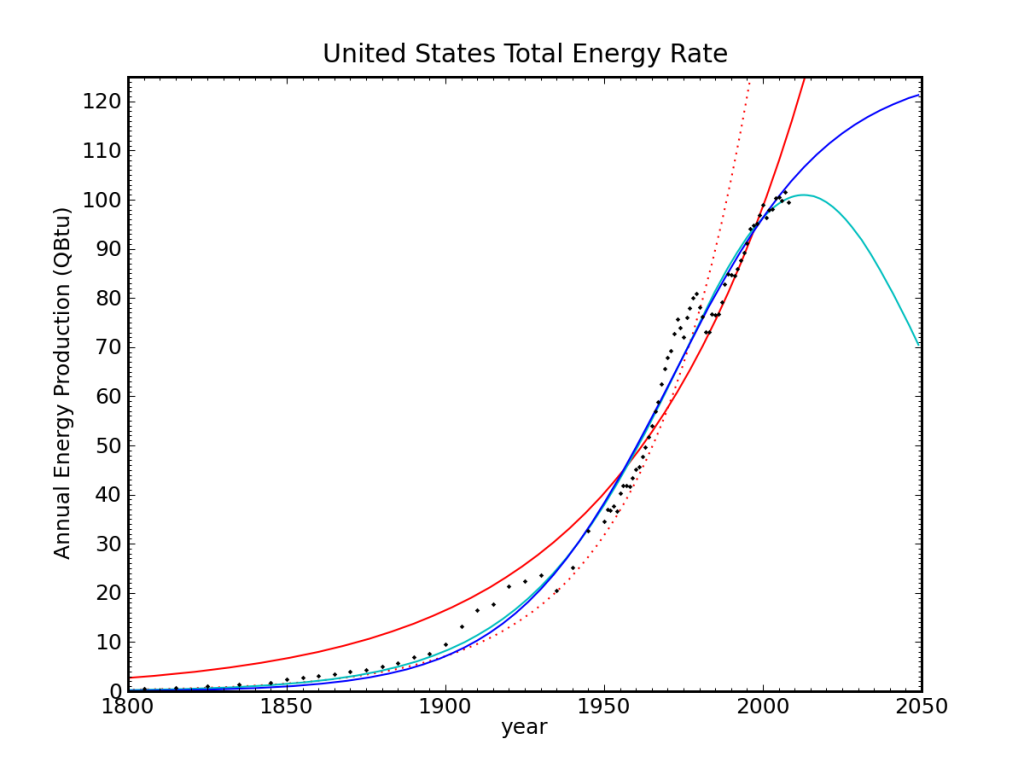
But let’s look at the problem in more familiar, linear space. The fit in log-space is dominated by the distant past, while all the interesting stuff is happening recently.
I conducted the same fitting procedure in linear space, as shown here. The red exponential curve scales back to 1.8% per year growth from 3.0%, and as such is muted at the top end—but poorly fits most of the data. The red dotted line is the fit from the logarithmic graph, which does a better job of fitting until about 1980. In this case, the blue sustainable trajectory has its half-way point (inflection) in 1971, and will top out at 127 QBtu per year (equivalent to 4.2 TW). The cyan finite resource curve peaks in 2013 at 101 QBtu/yr (3.4 TW). So the story is very similar to that of the log-space plot/fit.
Either logistic scenario is a radical departure from the indefinite growth scenario. As pointed out in the post on economic growth, even level energy—together with limits to efficiency improvement—spells trouble for continued economic growth. The finite resource scenario is downright disastrous.
I have already pointed out the serious fallacy of extrapolating the future based on some mathematical curve. So don’t mistake the message here: I’m not predicting the future, or favoring one curve over the other (except to point out that the straight-up exponential is the worst of the three by a fair margin). The reality is probably not well described by any of these curves. Physics and practical accomplishment, rather than imagination or a mathematical model, will decide the future. I for one am humbled by the fact that vastly different logistical scenarios do equally well at fitting the data to date. But I also recognize that we have based progress to date on a rapidly diminishing finite resource. Unless we deliberately plan for a renewable—and ideally sustainable—trajectory, acting decades ahead of the crunch, we won’t be happy with the result.
Views: 2934
I’m gobsmacked by how many seemingly smart people totally missed the point you’re hammering home again here. While I saw many busily trying to disprove what they saw as your preposterous prediction, I saw not one comment suggesting a global (or even local) economic model which is “sustainable” in a long-term negative growth environment. I have not seen any mainstream economist willing to front up on the idea of a world which is no longer “growing”. Someone come up with this magic pudding quickly, please. How do we maintain ~6 billion souls when every population and economy is contracting? What will that world require? What would that look like (in the best-case scenario)?
Brendan, you say: “every population and economy is contracting.” [Really? (edited)]
The population problem is a severe one because in a sum of exponentials, only the one with the largest exponent matters in the end. Thus you can have population contraction in the US, EU, Russia, Japan, and even China, and the non-contractors will make up for it. Amartya Sen, in Poverty and Famines made the point that the largest determinants of population growth are: (1) access to medical care; (2) the educational status of women. The problem is cultures can be hostile to these things, and thus a culture can perpetuate a high-growth rate even when surrounded by cultures that are not, and they could indeed export their culture.
To illustrate the sum of exponentials point, the current rate of population growth (1.14%) yields a population of one trillion in just 360 years. Even if 99% of the world were to stop growing, if one culture or nation continues to grow, it can soon overtake the others; for example, if Uganda continues its 2.69% growth rate, its population would increase from 32.4 million in 2009 to 457 billion (almost half a trillion) in 360 years. It will be necessary for the zero population growth policy to be global.
Look at the Figure 2 of the Annual Energy Report 2009 from the EIA and you will see that energy consumption (better called “power use”) peaked in 1978-79 and has declined since. The growth in power use has been because of population growth, which is slowing in the US.
“United States Total Energy Rate” does not seem to account for trade. If you import things that you used to manufacture or used to grow like capital equipment or food –alot of stuff in my house now says ‘made in China’ — you’re effectively importing and consuming embodied energy which doesn’t show up in the data above.
This argument has been used to propose shifting responsibility for some GHG emissions from producing countries to consuming countries.
All interesting from a science standpoint but the extension to economics is still seriously flawed. Energy usage per unit of GDP has been falling for decades, even in places like China.
http://nomoneynoworries.files.wordpress.com/2011/01/energy-use-per-unit-of-gdp.jpg
This is even more obvious from you data since GDP has been continuing to rise while energy use has flattened out.
While some of that might be due to increased efficiencies at using energy, I would be willing to bet almost all of it is due to increased leverage. GDP is in a sense “artificially inflated” by the amount of private and government debt, which are fueling what Mr. Murphy called in a previous post “sectors of economic activity not directly tied to energy use. Loosely, this could be thought of as non-manufacturing activity: finance, real estate, innovation, and other aspects of the ‘service’ economy.”
This graph from Gail the Actuary at Our Finite World shows what I’m talking about:
http://gailtheactuary.files.wordpress.com/2011/07/united-states-debt2.png
Essentially, the apparent growth in GDP has been borrowing against the confidence in future growth. But like many over-leveraged financial firms have discovered over the years, the actual returns (in this case GDP) do not necessarily reflect the underlying assets (in this case the actual productivity of the economy).
GDP is simply the market value of all final goods and services produced in a country in a given period. Services like heart surgeries aren’t valued fantastically more than goods like televisions because they take a proportional about of energy to produce. So the fact that energy production can’t increase exponentially for very long doesn’t imply that GDP can’t either.
This physics based limit on GDP is bogus.
Dave,
the energy cost per unit of GDP must go down when economies shift their growth to the tertiary sector. An office high-rise in the banking district of London or New York may generate as much GDP as a steel mill with much less (fossile) energy input.
Which is also the reason for the GDP and energy use curves diverging at some point. Yes, part of the declining ENERGY/GDP will be from greater efficiency and possibly better resource allocation in farming and industrial manufacturing but a lot of it will be because from increasing societal complexity and a growing service industry.
You can grow the GDP if you can convince people that posh haircuts cost $100. You can also convince people that marketing executives are on the whole doing a productive and useful service in convincing people to buy new cars every two years or so. But this service will be worthless and will disappear when there is little energy to grow food and heat houses.
This part of the GDP will just vanish. It´s a bit like a card house really. The top jobs in the complexity pyramid rely on the lower tiers to work and to generate a big enough share of the profit to be siphoned off by the higher tiers.
When the lower tiers – farming, industrial manufacturing, transportation – get into trouble because there is not enough energy to go around, the upper tiers will face a strong challenge to survive. They can survive for a while in an oppressive system (like George Orwells 1984 or USA 2011) but there is no long-term prospect of fooling physical laws. Which is what I love about this blog.
To give you a more down-to-earth example. I (being an exemplary economist :-)) have been teaching yoga for the last 10 years. I am doing a good service for people´s health and mental well-being and they in return are willing to pay me for my classes.
When prices for food and heating start to go up, what do you think will be the more important position in the family-ledger to spend money on: my yoga-classes or bread and a warm house?
A lot of people will start getting their workout from gardening and farming, which will do wonders for their physical and mental health and leave me on the lookout for a new job.
Food costs and other material necessities go up proportionally with the population, so of course if the population increases exponentially so must material costs and so the energy considerations become important.
But this is irrelevant to the GDP connection. For example the population could simply increase linearly. Then the energy needs would increase linearly. But neither of these constrains the GDP to grow linearly as the value of a good or service is not related to the magnitude of the energy inputs.
So the limit on exponential economic growth as put forward in the original posts are mistaken. There may be other limits but the energy considerations aren’t one of them.
The last statement says energy is irrelevant to economic growth. I just want to point out this not-too-uncommon belief so people can ponder on their own and see if it makes sense to them. If, after reflection, you think it’s correct, then you may be wasting your time reading a physics-based blog.
Hint: is it simply a coincidence that our explosion in innovation, technology, population, economic growth by chance aligns with our discovery of vast deposits of fossil fuel energy? Do you think the future will be governed by an entirely different set of rules/relationships?
I made no statement to the effect that “energy is irrelevant to economic growth.”
There was one that questioned the “limit on exponential economic growth” as put forward in the original post which focused on the impossibility of continued exponential increase in energy.
I contend that these are two entirely different things. The word “exponential” is not I think superfluous. If you can demonstrate otherwise please do so.
And of course I would agree that is it not “simply a coincidence that our explosion in innovation, technology, population, economic growth by chance aligns with our discovery of vast deposits of fossil fuel energy.” But that has nothing to do with the narrow point I am asserting.
Of course running out of energy dooms everything. But not being able to have 3% growth in energy every year has not been shown to imply that we can’t still have 3% or whatever growth in gdp every year.
If I am wrong fine, but please show your work. That would seem a rather mild request on a physics based blog.
If the energy sector and energy bounded sectors start to grow sub-exponentially and the non-energy sector continues to grow exponentially, after some amount of time the non-energy part will be many many orders of magnitude larger than the energy bounded sector.
Farming is a good example of an essential and energy bounded part, as it is very much a physical process. Let us pretend it is the only energy-bound sector.
Now consider the social consequences of this exponential growth scenario, under a non-exponential population [1] – food must become vanishingly cheap for those working in the non-energy sector, by virtue of the exponential separation between the GDP allocated to farming and that allocated to other activities. After sufficient exponential growth, a single nanosecond of activity in the non-energy sector will be sufficient to purchase all of the food made in a millenium. This circumstance is clearly absurd.
Did you even read the original post? It addressed exactly these questions.
No these questions were not really addressed in the first post “Galactic-Scale Energy”.
In the second post, “Can Economic Growth Last”, it got close to the point I was making but ended up with just a hand-waving comment “It is fantastical to think that an economy can unmoor itself from its physical underpinnings and become dominated by activities unrelated to energy, food, and manufacturing constraints.”
I am simply pointing out the economy doesn’t have to completely “unmoor itself from its physical underpinnings” to still grow exponentially.
I think I understand the confusion. You maintain that exponential economic growth can continue, even if still tied at some level to the physical world (thus physics). If the latter is finite (picture Earth), or even rate-limited then the degree to which the exponentially growing economy depends on the finite or saturated resource must steadily diminish. For this to go on indefinitely would constitute an essentially complete detachment. The physical part would be relegated to a negligibly small part of the total economy (1%, 0.1%, 0.01%, etc. as far as you like).
This is far more than hand-waving, and is mathematically solid. Exponential growth is a ruthless partner. Anything that sees limits to growth is fundamentally incompatible in the end, and a complete divorce is mandated. I see a physical world of finite resources and do not question that our rate of energy use must eventually flatten or decline (and on a relevant timescale). I believe it is possible for economic growth to continue for some time after, but for only a while at best.
I don’t think the observation that we will eventually run out of energy resources if we keep consuming them is a particularly novel one.
Your important/excellent contribution was to point out that our current exponential consumption of them would run them out really fast. In a mere 1350 years for example, you had us sucking up the entire output of the sun. So obviously at some point our rate of consumption of these is going to have to slow down.
The question here is does that mean the GDP must necessarily slow down? I don’t see that it does. Of course I agree that this means that what you are calling the physical part would be “relegated to a negligibly small part of the total economy”. But one could make the same argument about the world production of salt. Something that is very necessary and at one time very valuable in even small quantities. Now it is so cheap we take it for grated, yet without salt production we’d all die. There would be no be no economy. But whether it makes up “(1%, 0.1%, 0.01%, etc. as far as you like).” of the economy doesn’t matter. The ratio just has to be greater than zero. Likewise no matter how small the physical part of the economy may be it will never be irrelevant. So there is never a “complete detachment”. Magnitude matters, not ratio.
Economic value is much more mental than physical and that once you start getting past basic physical requirements for life the importance of the psychological aspects start to dominate. The evidence seems clear on this as there is little correlation between the cost of the energy inputs of a product and the price of the product itself other than in the setting of a lower bound. Then we have virtual products/devices that require essentially no energy but the activity of the mind. I don’t think there are any clear, impossible limits to this that you can point to like the capturing of the output of the sun. Or at least no such limits have been shown as you did for energy.
You should see this as good news, because it means we can limit the growth of our energy without necessarily limiting the growth of the economy, only the direction and composition.
Dave: A well-articulated point. Your point is that, like salt, once we have enough energy to meet our basic needs, it need not grow more (let’s say under a stabilized population) in order to keep the economic growth engine humming. And I can think of other examples of vital resources that have extraordinarily high marginal value, but becomes very cheap once we have enough. Water is one.
But I have to put energy in a different category. It’s the capacity to do work—the lifeblood of activity. When energy (and related efficiency gain) saturates, so must mining, manufacture, agriculture, transportation, and a host of other physical activities. For the economy to keep growing in the face of physical stagnation would require activities of ever (incessantly) diminishing energy intensity. None of our current economic activity has zero energy intensity (if done by humans, we have to eat, and have finite mental capacities and available time). So I think there really is a residual physical limit than can be pressed. But before we even get to that, I think the increasingly “mental” economic activity you describe is just not going to become 99.9% (and up) of economic activity. Many (think outside of our nerd box) want “stuff,” not “fluff.” I point again to the non-coincidental surge in tech/pop/economy that accompanied our discovery and profligate use of fossil fuel energy. Some decoupling is clearly demonstrated/possible, but let’s not get carried away with that abstraction. Energy will always be a foundational necessity for economic activity.
[Edited down to essential points…]
You have ably demonstrated that if human civilisation’s energy usage were to increase exponentially it would soon hit physical barriers. However, it is false to therefore conclude – as you and some of the contributors to this comments section have – that economic growth consequently has fundamental limits.
The root of that misunderstanding is that you are comparing a real physical thing (the energy usage of human civilisation) with a subjective social construct (global GDP). It’s true that for the entirety of recent history, GDP growth has been closely (although not precisely) correlated to energy use, but the two are not intrinsically linked.
It is entirely possible to imagine a scenario where planet of humans run a civilisation on a planet in which all their physical artefacts (computers, transport systems, household appliances etc) become continuously more cunning, efficient and cleverly designed, while shrinking in terms of entropy conversion. Such a civilisation would have a sustainable energy-use profile, even while increasing when measured in terms of that made-up quantity – real nowhere else but in human minds – that we call ‘money’.
A number of folks have made similar points: that GDP need not be tied to the physical world. It’s an attractive thought: I do understand the intellectual appeal of everlasting efficiency improvements and of infinite decoupling. But the economic growth post dealt with the fact that we can’t just keep squeezing efficiency with no limit in sight. We humans are ruthless extrapolators, so that we take the fact that we can make money off less energy intense activities (but never zero energy) to mean that we can carry this to an absurd extreme.
To keep GDP growth indefinitely on a fixed energy diet means that anything requiring energy becomes an ever-smaller part of GDP, until it carries negligible value. It won’t work, no matter how pure and attractive the thought is. There is plenty of proof for lower energy intensity economic activities, but no proof that we could A) reduce energy intensity to zero, and B) base 100% of our economy on such activities. Both are required for indefinite GDP growth on a fixed energy income. Not. Gonna.
I disagree with your last comment.
David Beckham uses exactly the same amount of energy as a footballer who plays in the second division of Britain’s soccer leagues, but who gets paid 5% of his salary, and yet Mr Beckham’s makes a much larger blip on the UK’s GDP profile. The energy-intensity difference between Mr Beckham and this other footballer is zero. The GDP difference is larger by a factor of 20.
What you have certainly demonstrated is that the nature of tomorrow’s economy will be profoundly different from that of today – just one of the many things about the future that makes it so exciting.
I think my point was not clear enough, and there is a misunderstanding about the term “energy intensity.” In your example, Beckham’s energy intensity is 1/20 that of the average bloke per unit of GDP. Show me an athlete who can accomplish Beckham’s payscale without expending any energy, and I’ll say you won: we have a zero intensity situation with perfect decoupling. It’s very easy to find examples of low energy intensity. But A) this does not mean it can become arbitrarily low, or B) that we can accomplish arbitrarily low energy intensity across the board. It is not sufficient to find outlier examples and claim that the future will have nothing but such outliers—and even more exceptional than the best of the best today. Nice dream, though. It would be an exciting version of the future.
In order to have indefinite economic growth on a finite energy backdrop requires a push toward zero energy intensity across the board for what amounts to 100% of economic activity. I sense an end of progress on this exchange, so will not burden the readers with more of the same.
I’ve enjoyed your blog and linked to it from mine (http://hamiltonianfunction.blogspot.com). I’ve touched on quite a few things that intersect your topic space, you might be interested spending a minute or two at:
total energy use in my family;
carbon footprint adventures;
going renewable part 1; and
going renewable part 2.
Quoting our ex-governator (whom I’ve seen at arpa-e giving a speech on renewable energy), “Ah’ll be bach.”